The CODAR system needs to compute three components in order to measure currents (and other properties).  These are the velocity of incoming waves (each CODAR site measures one radial comment), distance to the waves, and the angle the waves are traveling relative to the CODAR site.

Like other HF radar systems, CODAR velocity measurements are based on a concept known as "Resonant Bragg Scattering".   The signal sent from the CODAR antenna has a known frequency (currently 25 MHz for the system at Rutgers). Since  we know that the signal is moving at the speed of light we can determine the wavelength of the signal to be wavelength = speed of light / frequency which results in a wavelength of approximately 12 m.

CODAR uses the principal of resonant theory to take advantage of Bragg Scattering to maximize the scattered HF signal.  Resonance will only occur for a given wavelength:
 
 

Since the CODAR antennas are place at basically sea level the incident angle of the signal is assumed to be zero. Therefor the equation reduces to:
 
 

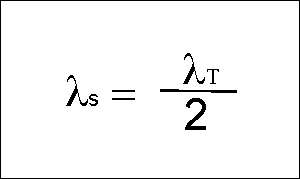
When the signal hits waves with wavelength equal to 1/2 the transmitted wavelength,  the return signals which are scattered back to the antenna will be in phase.  This results in a very strong signal for waves with wavelength equal to 1/2 the transmit wavelength.
 
 

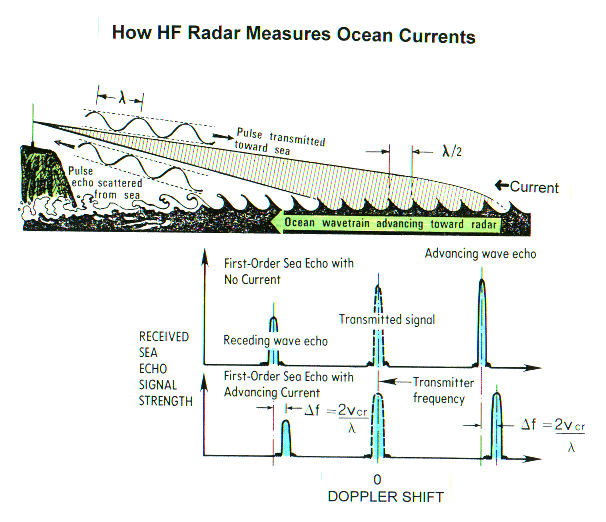
Since the CODAR system measures the backscattered signal, the current speed can be extracted by determining the Doppler Shift of the waves.  The above equations assume that the reflecting waves are not moving. Because the waves are moving, the frequency of the signal returned is not quite the same frequency as the signal transmitted.  Waves moving toward the receiver increase the return frequency, while waves moving away decrease the return frequency.  The magnitude of the frequency shift can be determined by the following equation:
 
 

The equation above assumes that there is no surface currents changing the motion of the waves. If there is a  surface current there will be an additional Doppler shift which will vary with the magnitude and direction (radial) of the current. By using the additional measured frequency shift and the Doppler equation we can compute the velocity of the component of the current moving towards are away from the CODAR unit.
 
 

Now that we have calculated the current velocity we have to determine the range to target and the angular direction to the target.  CODAR uses a frequency modulated signal, which has frequency that increases linearly with time. The time delay, which can be used to determine the range to target, can be measured by subtracting the return signal from the transmitted signal.   CODAR is a "direction finding system" which uses the  "MUSIC" algorithm (which stands for MUltiple SIgnal Classification) to computed signal bearings every 5°.  The signal is received by two loop antennas and a monopole which form a beam pattern (see figure below).  The signal the monopole receives does not vary with the direction of the incoming signal, while the signal received by the two loop antennas, positioned at a 90° angle, varies with direction.  This information is used by the software to determine the direction of the signal.
 
 

Now that we have calculated the radial velocity of currents, distance to target, and the angle to target we can construct current vector maps for each of our two CODAR sites.
 
 

For the area which vector data from the two CODAR sites overlap, we can calculate the velocity and direction of the current:
 
 

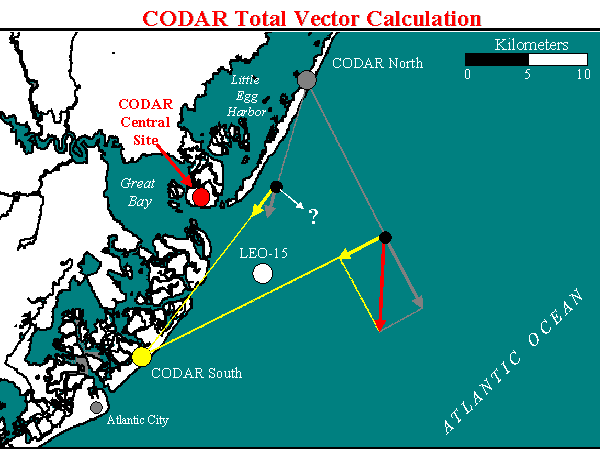
Below is an example of total current vector map for the LEO-15 area derived from CODAR data (plotted over satellite derived sea surface temperature).  In this map vectors represent the average of data available from a circle with a diameter of  2.5 kilometers.