Abstract
Introduction
What is CODAR?
Current Measurement
Wave Measurement
How Do CODAR Measurements Compare to Data From Other Platforms?
What Can This Data Be Used For?
Conclusion
References

Introduction

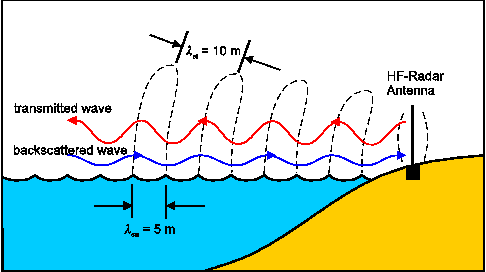
The high frequency (HF) band of the electromagnetic spectrum, with frequencies of 3 to 30 MHz corresponding to wavelengths of 100 to 10 m, is within the radio bands.  Crombie (1955) found that some HF signals recorded near the ocean had a Doppler shift of less than a hertz above and below the transmitted signal and concluded the shift was due to "Bragg scattering" of the signal by ocean waves.  Bragg scattering occurs when the transmitted energy (HF radar waves in our case) is reflected by ocean waves that are traveling radially towards or away from the radar and whose wavelength is half as long as the transmitted signal.  These reflections result in strong peaks in the backscatter spectrum (Figure below).  HF radars use the scattering of the first order Bragg peaks to estimate ocean currents by comparing the scattering to the expected Doppler shift due to the Bragg waves (additional shift is then attributed to currents).

What is CODAR?

how is it different from Phased array?
 
 
 
 

Current Measurement

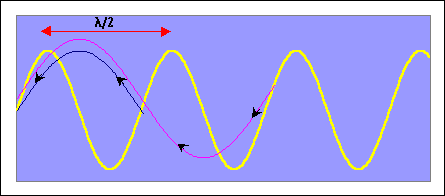
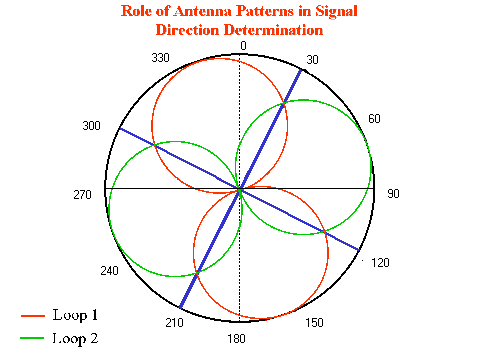
CODAR measurements are based on a concept known as "Resonant Bragg Scattering".   The signal sent from the CODAR antenna has a known frequency (currently 25 MHz for the system at Rutgers).  Since  we know that the signal is moving at the speed of light, we can determine the wavelength of the signal to be:

When the signal hits waves with wavelength equal to 1/2 the transmit wavelength,  the return signals scattered back to the antenna will be in phase.  This results in a very strong signal for waves with wavelength equal to 1/2 the transmit wavelength.
 
 

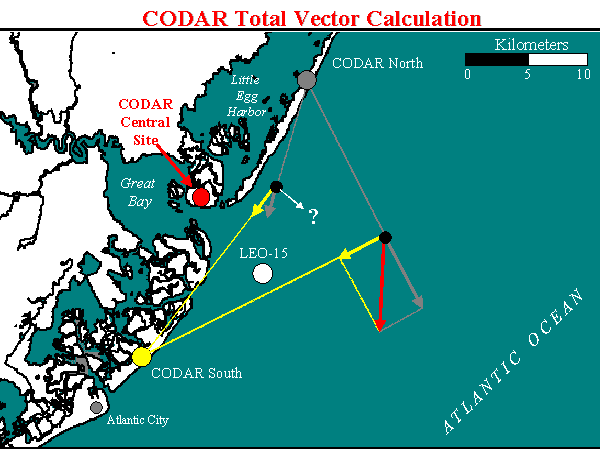
Once the CODAR system measures the backscattered signal, the current speed can be extracted by determining the Doppler Shift of the waves.  Because the waves are moving, the frequency of the signal returned is not quite the same frequency as the signal transmitted.  Waves moving toward the receiver increase the return frequency, while waves moving away decrease the return frequency.  The magnitude of the frequency shift can be determined by the following equation:

{get eqn from Josh}

Since we know the wavelength of the waves that scattered the signal, we can use the dispersion relation to determine the speed of the waves:
 

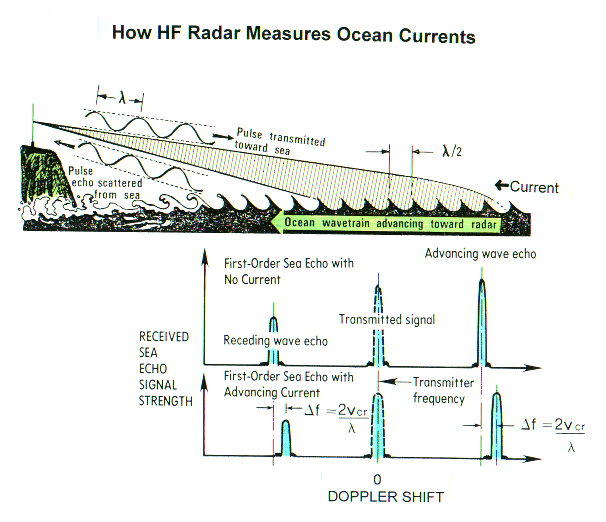
These calculations give us the Doppler Shift of the signal assuming there is no current.  The current velocity is then simply the difference between the measured frequency shift and the doppler shifted frequency calculated above.

Significant wave height is the estimated average height of the highest one-third of the waves.
 

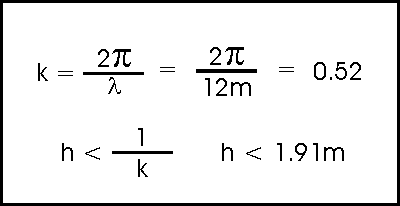
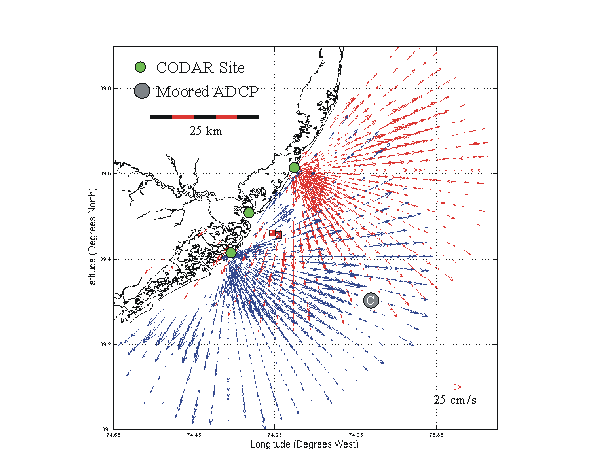
How Do CODAR Measurements Compare to Data From Other Platforms?
 
 

Conclusion
 

References